Heap vs Binary Search Tree (BST)

In this post, we will learn the difference between Heap and Binary Search Tree (BST) in Java. This is a frequently asked question in Java interviews for beginners. Let's dive into it.

Difference between Heap and Binary Search Tree (BST) in Java

Feature Heap Binary Search Tree (BST)
Structure Heap is a specialized tree-based data structure where the key of each node is either greater than or equal to (max heap) or less than or equal to (min-heap) the keys of its children. BST is a binary tree where the left child of a node has a key less than the node's key, and the right child has a key greater than the node's key.
Balancedness Heaps are usually not balanced; they are binary trees with some specific ordering properties. BSTs can be balanced or unbalanced, depending on the order of insertions and deletions. Balanced BSTs (e.g., AVL trees, Red-Black trees) maintain height balance for efficient operations.
Main Operations Heap supports efficient insertion, deletion (extract-max or extract-min), and finding the maximum or minimum element in O(log n) time complexity. BST supports efficient search, insertion, and deletion operations in O(log n) time complexity for balanced trees. For unbalanced BSTs, the time complexity may degrade to O(n) in the worst case.
Use Cases Heaps are commonly used in priority queues and heap-based sorting algorithms like Heap Sort. BSTs are used for fast searching and dynamic data sets where elements are inserted and removed frequently, such as in database indexing and symbol tables.
Implementation Heaps are usually implemented as arrays with heapification algorithms to maintain the heap property. BSTs are implemented using linked data structures (nodes with left and right pointers) or array representation (e.g., array-based BSTs).
In-order Traversal Heap does not guarantee in-order traversal; it only satisfies the heap property. BST's in-order traversal visits the nodes in ascending order (sorted order) if the tree is balanced.
Time Complexity for Searching Heap has O(n) time complexity for searching an element as there is no specific order among the elements in each level. Balanced BST has O(log n) time complexity for searching, as it halves the search space in each step.
Heap Property The heap property ensures that the root node has the highest (max heap) or lowest (min-heap) key among all the nodes in the heap. BST property ensures that the left subtree of a node contains keys less than the node's key, and the right subtree contains keys greater than the node's key.
Example 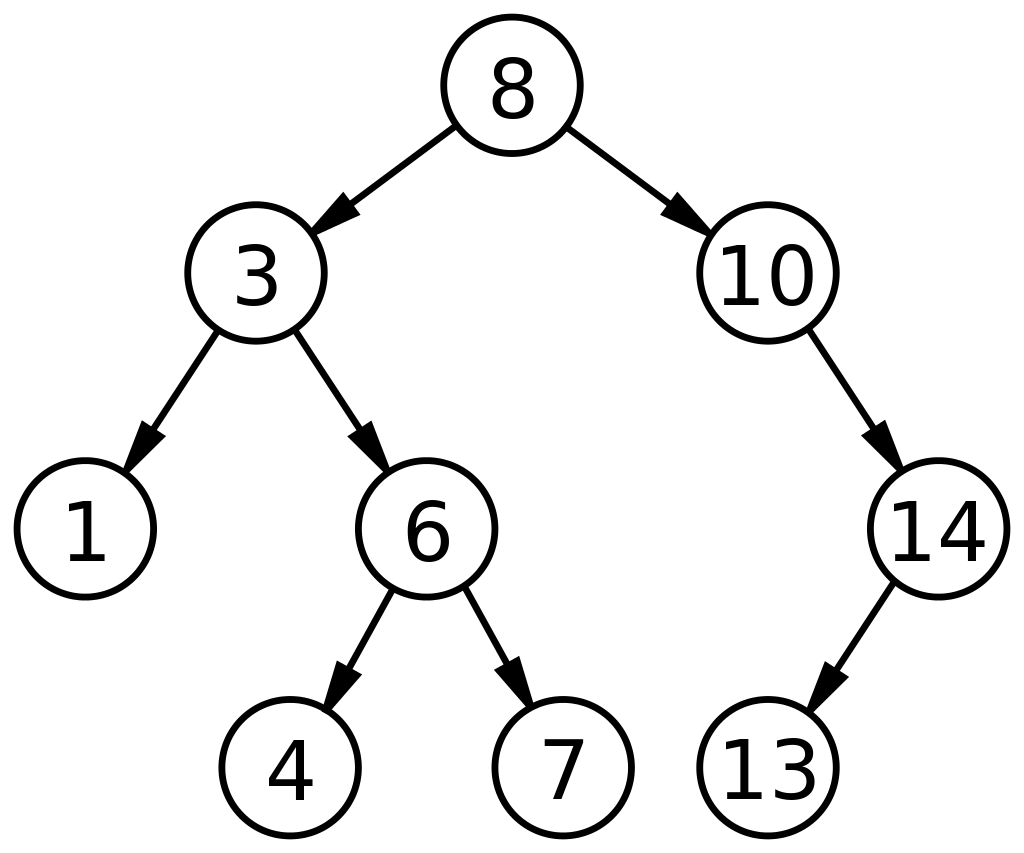Heap Example BST Example
In summary, heaps are specialized tree-based data structures with specific ordering properties, used mainly for priority queues and heap sorting. On the other hand, binary search trees are general-purpose binary trees with elements ordered based on their keys, used for efficient searching, insertion, and deletion operations. The choice between a heap and a BST depends on the specific requirements of the application or problem at hand.


Related Java Source Code Examples:

Java Record Class Example Java Text Blocks Example Java Record Builder Pattern Example Java Record Constructor Example Java Record Constructor Validation Example Java Record Serializable Example Java Record Getter Example Java Record Default Constructor Java Records CRUD (Create, Read, Update, Delete) Example Java Record Custom Methods Java Record Custom toString Java Record Custom equals and hashCode Java Records with Spring Boot CRUD Example Java Record Implement Interface Example Java Switch Expressions Example Java Pattern Matching for instanceof Java Sealed Class Example Java Sealed Class vs Final Class Java Sealed Class Record Example Java Sealed Class Generic Example Java Sealed Class Enum Example Java Sealed Class Switch Example Java var Keyword Example Java CompactNumberFormat Class Example Java Vector API Example Pattern Matching for Switch Example Collectors.teeing() in Stream API Example Java FileSystems.newFileSystem() Method Example Java Files.readString() and Files.writeString() Example Java String isBlank Example Java String strip Example Java String repeat Example Java String lines Example Java String stripIndent Example Java Binary Search (Recursive and Iterative) Program Java Happy Birthday Program Java Program That Calculates the Age of a Person Java Logical And Or Xor Examples Java Format Date and Time Examples Java Convert String to Date and Time Java Convert Roman to Integer Number Java Convert Octal to HexaDecimal Java Convert Octal to Decimal Java Convert Integer Number to Roman Number Java Convert Hexadecimal to Binary Java Convert Decimal to Octal Java Convert Decimal to Binary Java Convert Binary to Decimal Java Convert Binary to an Octal Java Convert Binary to a Hexadecimal Java Convert a Unix Timestamp to Date Time Java Check If Two Integer Arrays are Equals Java program that removes all white spaces from the given string Java 8 program that counts the number of vowels and consonants in a given string Java 8 program that reverses words of a given string Java Program to Count Number of Digits in an Integer Java Program Fibonacci Series Using Recursion Java Program to Find Factorial of a Number Java program to calculate the area of Triangle Java Program to Calculate Area of Square Java Program to Calculate Area of Rectangle Java Program to find the Smallest of three numbers using Ternary Operator Java Program to Find Largest of Three Numbers Java Program to Find GCD of Two Numbers Java Program to Check Armstrong Number Java Program to Generate Random Number Java Program to Check if Number is Positive or Negative Java program to check prime number Java Program to Calculate Simple Interest Java Program to Swap Two Numbers Without using a Temporary Variable Java Program to Swap Two Numbers Java Program to Find ASCII Value of a Character Java Program to Check Whether an Alphabet is Vowel or Consonant Java Program to Check Leap Year Java Program to Check Even or Odd Number Java Program to Add Two Numbers Java Program to Swap Two Strings Without Using Third Variable Java Program to Swap Two Strings with Third Variable How to Get All Digits from String in Java Find Duplicate Number in Array in Java How to Get Current Working Directory in Java? Check Palindrome String in Java Java Program to Create Pyramid Of Numbers Create a String object in Java Example Java String valueOf() Method Example Java String.trim() Method Example Java String.toUpperCase() Method Example Java String toLowerCase() Method Example Java String toCharArray() Method Example Java String substring() Method Example Java String.startsWith() Method Example Java String split() Method Example Java String.replaceFirst() Method Example Java String.replaceAll() Method Example Java String replace() Method Example Java String regionMatches() Method Example Java String length() Method Example Java String lastIndexOf() Method Example Java String intern() Method Example Java String indexOf() Method Example Java String getChars() Method Example Java String getBytes() Method Example Java String equals() and equalsIgnoreCase() Example Java String.endsWith() Method Example Java String.contentEquals() Method Example Java String.contains() Method Example Java String concat() Method Example What's the Simplest Way to Print a Java Array? Merge two String Arrays Example Checks If a String is Not Empty and Not Null in Java Reverse a String in Java Using StringBuilder Capitalize the First Letter of a String in Java Example Java String codePointAt() Method Example Java String Compare Using equals() Method Sort String in Descending Order in Java Sort String in Ascending Order in Java How to Get All Digits from String in Java Java String getDigits() Utility Method Java String isAlphanumeric() Utility Method Java String isAlpha() Utility Method Java String countMatches() Utility Method Java String mergeStringArrays(String array1[], String array2[]) Utility Method Example Java String quote( String str) Utility Method Java arrayToMap(String[][] array) Utility Method Java String fromBufferedReader() Utility Method - Converts a BufferedReader into a String Java String isNumericSpace() Utility Method Java String isNumeric() Utility Method Java String isAlphanumericSpace() Utility Method Java String isAlphaSpace(() Utility Method Java trimArrayElements() Utility Method Java trimArrayElements() Utility Method Java String containsWhitespace() Utility Method Java String isBlank() Utility Method Java String isAnyEmpty() Utility Method Java String isNotEmpty() Utility Method Java String isEmpty() Utility Method Java String Uppercase First Character Example Java String uppercaseFirstChar() Utility Method Java String trimTrailingCharacter() Utility Method Java String trimLeadingCharacter() Utility Method Java String trimTrailingWhitespace() Utility Method Java String trimLeadingWhitespace() Utility Method Java String trimAllWhitespace() Utility Method Java String trimWhitespace() Utility Method Java StringBuilder trimToSize() Method Example Java StringBuilder substring() Method Example Java StringBuilder setLength() Method Example Java StringBuilder reverse() Method Example Java StringBuilder lastIndexOf() Method Example Java StringBuilder insert() Method Example Java StringBuilder indexOf() Method Example Java StringBuilder getChars() Method Example Java StringBuilder ensureCapacity() Method Example Java StringBuilder deleteCharAt() Method Example Java StringBuilder delete() Method Example Java StringBuilder codePointBefore() Method Example Java StringBuilder codePointAt Method Example Java StringBuilder capacity() Method Example Java StringBuilder append() Method Example Java Convert Integer to String Example Java Convert Float to String Example Java Convert Double to String Example Java Convert Short to String Example Java Convert Long to String Example Java Convert Character to String Example Java Convert Byte to String Example Java Convert Boolean to String Example Java Convert String To Short Example Java Convert String To Long Example Java Convert String To Integer Example Java Convert String To Float Example Java Convert String To Double Example Java Convert String To Byte Example Java Convert String To Boolean Example Clear ArrayList in Java Example Java ArrayList add(), get() and set() Method Example Iterate over ArrayList Using forEach Java Iterate over ArrayList using Iterator in Java Java ArrayList indexOf() and lastIndexOf() Example Search an Element in an ArrayList in Java Java ArrayList removeAll() Method Example Java ArrayList remove() Method Example How to Iterate over ArrayList in Java How to Remove Element from ArrayList in Java How to Access Elements of ArrayList in Java Create ArrayList from Another ArrayList in Java How to Create an ArrayList and Add Elements to It Java ArrayList subList() Example Get Index of Elements in ArrayList Example Java ArrayList removeIf() Example Copying part of Array in Java Example Sorting an Array using Arrays.sort() Method Check Two Arrays are Deeply Equal Java Arrays.copyOf() Example - Copying an Array Java Arrays.binarySearch() Example - Searching an Array Java Arrays.asList() Example - Convert Array to ArrayList Sort String in Ascending Order in Java Sort String in Descending Order in Java Sort Integer in Ascending Order in Java Example Sort Integer in Descending Order in Java Example Sort User-Defined Objects in Ascending Order in Java Sort User-Defined Objects in Descending Order in Java Sort User-Defined Objects using Comparator Interface Java LinkedList add(), addFirst(), addLast() and addAll() Example Iterate LinkedList using forEach() Method Example Iterate through a LinkedList using an Iterator in Java Iterate or Loop Through Elements LinkedList in Java Example Search Elements of LinkedList in Java with Example Remove First and Last Elements of LinkedList in Java Get Elements from LinkedList Java Example Java LinkedList remove(), removeFirst(), removeLast(), removeIf() and clear() Example Create HashSet in Java Iterating over a HashSet using Iterator Iterate over HashSet in Java Remove Element from HashSet in Java Create HashSet in Java Iterating over a LinkedHashSet using forEach() Method Example Iterate over LinkedHashSet using Iterator in Java Example Iterate over LinkedHashSet in Java Example Remove all Elements from LinkedHashSet in Java Example Remove Element from LinkedHashSet Example Check If an Element Exists in Java LinkedHashSet Example Create LinkedHashSet in Java Example Create TreeSet in Java Example Iterating over a TreeSet using Java 8 forEach() Method Iterate over a TreeSet using Iterator in Java Example Iterate over TreeSet in Java Example Remove All Elements from Java TreeSet Example Remove First and Last Element from TreeSet in Java Remove Element from TreeSet in Java Example Check If an Element Exists in Java TreeSet Example Java TreeSet add(), first() and last() Method Example Java Convert ArrayList to Stack Example Java Convert Array to Stack Example Java Stack push() Method Example Java Stack empty() Method Example Java Stack peek() Method Example Java Stack search() Method Example Java Stack pop() Method Example Java Deque and ArrayDeque Examples Iterating over a Queue in Java Example Java Queue isEmpty(), size(), element() and peek() Example Java Queue Interface Example Java Priority Queue of User Defined Objects Example Java Create a Priority Queue with a Custom Comparator Create a Priority Queue in Java Example HashMap Stream and Filtering Example Java HashMap Iteration over Values Example Java HashMap Iteration over Keys Java HashMap replace() Example Java HashMap size Example Iterate over a HashMap Java using Lambda Iterate over a HashMap Java 8 forEach Iterating over a HashMap using Iterator in Java Iterate over HashMap in Java How to Store Employee Objects in HashMap Remove Entry from HashMap Java Check If Value Exists in HashMap Java Example Check If Key Exists in HashMap Java Example Create a HashMap in Java Example How to Delete Data from MySQL Table using Java How to Insert Data in MySQL Table using Java How to Create Database Table in Java JDBC DriverManager Example JDBC PreparedStatement Example JDBC Statement Example Java MySQL DataSource Example Update Query in MySQL using Java PreparedStatement Login Example with JSP + Servlet + JDBC + MySQL Database JSP + JDBC + MySQL Example Tutorial JSP Form Example with JDBC and MySQL Database Java JDBC CRUD Example - SQL Insert, Select, Update, and Delete Examples Java Swing JDBC MySQL Database Example Java JDBC Connection to HSQLDB Database JDBC Connection to H2 Database Example JDBC Connection to PostgreSQL Example JDBC Connection to Oracle Database Example JDBC Transaction Management Example JDBC Delete Query Example JDBC Select Query Example JDBC Update Query Example JDBC Insert Multiple Rows Example JDBC Create a Table Example What are different types of JDBC Drivers Advantages of Hibernate Framework over JDBC Java PostgreSQL Example Java H2 Insert Record Example Java H2 Embedded Database Example Java H2 Create Table Example Java Simple Lambda Expression Example Java Lambda Expressions Multiple Statements Java Lambda Expressions forEach Example Java Lambda Expression with Multiple Parameters Example Java Lambda Expression Runnable Example Java Lambda Expression Comparator Example Refactoring Factory Design Pattern with Lambdas Refactoring Chain of Responsibility Pattern with Lambdas Refactoring Observer Design Pattern with Lambdas Refactoring Strategy Design Pattern with Lambdas Iterate HashMap with forEach() + Lambda Expression Example Iterate HashSet with forEach() + Lambda Expression Example Iterate ArrayList with forEach() + Lambda Expression Example Java method reference to a constructor example Java method reference to static method example Java method reference to an instance method of an object example Java method reference to an instance method of an arbitrary object of a particular type Java 8 - Create Stream Object from Arrays Example Java 8 Creating a Stream from an Array Java 8 - Create Stream Object from Collection, List, Set Java 8 Stream - Sort List of String objects in Descending Order Example Java 8 Stream - Sort List of String objects in Ascending Order Example How to convert a Java 8 Stream to an Array? Java 8 Stream - allMatch(),anyMatch() and noneMatch() Example Java 8 Stream - Sort List of Objects by Multiple Fields Java 8 Stream - Sort List of Custom Objects in Ascending and Descending Order Java 8 - Stream filter(), forEach(), collect() and Collectors.toList() Example Java Stream filter null values example Java Stream filter map by values example Java Stream filter map by keys example Java 8 Collectors.counting() Example Java 8 Collectors.averagingDouble() Example Java 8 Collectors.toSet() Example Java 8 Collectors.toList() Example Java 8 Collectors.joining() example Java 8 Stream multiple filter operations Java 8 Stream filter map by keys Java 8 Stream filter map by values Java 8 Stream collect to list example Java 8 Stream Collectors.counting() exmaple Java 8 Collectors.collectingAndThen() example Java 8 Collectors.toList() Example Java 8 Collectors.minBy() and Collectors.maxBy() example Java Stream filter() Example Java Stream map() Example Java Stream flatMap() Example Java Stream distinct() Example Java Stream limit() Example Java Stream peek() Example Java Stream anyMatch() Example Java Stream allMatch() Example Java Stream noneMatch() Example Java Stream collect() Example Java Stream count() Example Java Stream findAny() Example Java Stream findFirst() Example Java Stream forEach() Example Java Stream min() Example Java Stream max() Example Java Stream reduce() Example Java Stream toArray() Example Java Function Functional Interface Example Java Supplier Functional Interface Example Java Consumer Example Java BiConsumer Example Java BiFunction Example Java Predicate Example Java 8 LongConsumer Example Java 8 IntConsumer Example Java 8 DoubleConsumer Example Java StringJoiner Example Create Optional Class Object in Java - empty(), of(), ofNullable() Methods Optional get() Method - Get Value from Optional Object in Java Optional isPresent() Method Example Optional orElse() Method Example Optional orElseGet() Method Example Optional orElseThrow() Method Example Optional filter() and map() Method Examples Java 8 program that counts the number of vowels and consonants in a given string Java 8 program that reverses words of a given string Java 8 - Copy List into Another List Example Check Whether the Given Year is Leap Year or Not in Java Java 8 forEach() Array Example Java 8 forEach() Map Example Java 8 forEach() Set Example Java 8 forEach() List Example Getting current date and time with Clock Convert LocalDate to Date in Java Convert LocalDateTime into Date in Java Connvert java.util.Date to java.time.LocalDateTime Example Convert java.util.Date to java.time.LocalDate Example Duration Between Two LocalDate in Java Java Convert ZonedDateTime to String Example Convert String to ZonedDateTime in Java Compare ZonedDateTime Objects in Java How to Get Hour, Minute, Second from ZonedDateTime in Java Convert ZonedDateTime to LocalTime Example Convert ZonedDateTime to LocalDateTime in Java Java ZonedDateTime now() and of() Method Example Convert LocalDateTime to LocalTime in Java Convert LocalDateTime to LocalDate in Java Compare LocalDateTime Objects in Java Java LocalDateTime - Get Hour, Minute and Second How to Get Day Month and Year from LocalDateTime in Java Java LocalDateTime now() Method Example How to Get Current Date Time in Java Convert LocalDate to String in Java Convert String to LocalDate in Java Java LocalDate isLeapYear() Method Example How to Get Number of Days from Month and Year using LocalDate in Java Compare Java LocalDate Objects Example How to Add Days Weeks Months and Years to LocalDate in Java How to get Day Month and Year from LocalDate in Java Java LocalDate now() Method Example - Get Current Date and Specific Date Convert LocalTime to String in Java Convert String to LocalTime in Java Java LocalTime isBefore() and isAfter() Method Example How to Compare Time in Java Java 8 - LocalTime getHour(), getMinute(), getSecond() and getNano() Example Java LocalTime now() Method Example - Get Current Time and Specific Time Java 8 Get Local Date Time in All Available Time Zones How to get year, month, day, hours, minutes, seconds and milliseconds of LocalDateTime in Java 8? How to get year, month, day, hours, minutes, seconds and milliseconds of Date in Java? Difference between Two Dates in Java Create LocalDateTime from LocalDate and LocalTime in Java

Comments

Post a Comment